Equation

This term is defined as the area under the plasma concentration versus time curve during the dosing interval at steady state divided by the dosing interval.

Thus:-

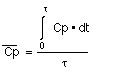
Equation XV-9 Average Cp for a Dosing Interval at Steady State

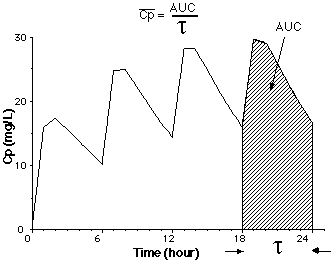
Figure XV-3, Plot of Cp versus Time after Multiple Oral Administration showing AUC at Steady State

By integrating the equation for plasma concentration at the plateau, between t = 0 and t = t gives:-

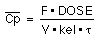
Equation XV-10 Average Cp for a Dosing Interval at Steady State

An interesting result of this equation is that we get the same average plasma concentration whether the dose is given as a single dose every t dosing interval or is subdivided into shorter dosing intervals.

For example 300 mg every 12 hours will give the same average plasma concentration as 100 mg every 4 hours. Of course, the difference between the maximum and minimum plasma concentration will be larger in the case of the less frequent dosing.

For example F = 1.0; V = 30 liter; t_1/2 = 6 hours or kel = 0.693/6 = 0.116 hr^-1.

We can now calculate the dose given every 12 hours required to achieve an average plasma concentration of 15 mg/L.

=

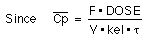
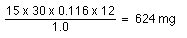
We could now calculate the loading dose

R = e^{-kel * [[tau]]} = e^{-0.116 x 12} = 0.25

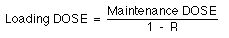
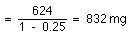
To get some idea of the fluctuations in plasma concentration we could calculate the Cpmin value.

Assuming that ka >> kel and that e^{-ka * t} --> 0, using Equation XV-8.

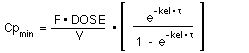
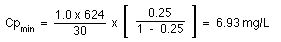
Therefore the plasma concentration would probably fluctuate between 7 and 23 mg/L (very approximate) with an average concentration of about 15 mg/L. [23 = 15 + (15-7), i.e. high = average + (average - low), very approximate!].

As an alternative we could give half the dose, 312 mg, every 6 hours give:-

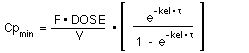
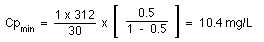
The 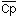would be the same

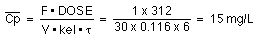
Thus the plasma concentration would fluctuate between about 10.4 to 20 with an average of 15 mg/L.

Copyright 2001 David W.A. Bourne